Parabolas

Return to MAT 130 main page

Go to Preview Activity

---

Textbook Reference: Section 10.3 The Parabola

---

Parabolas

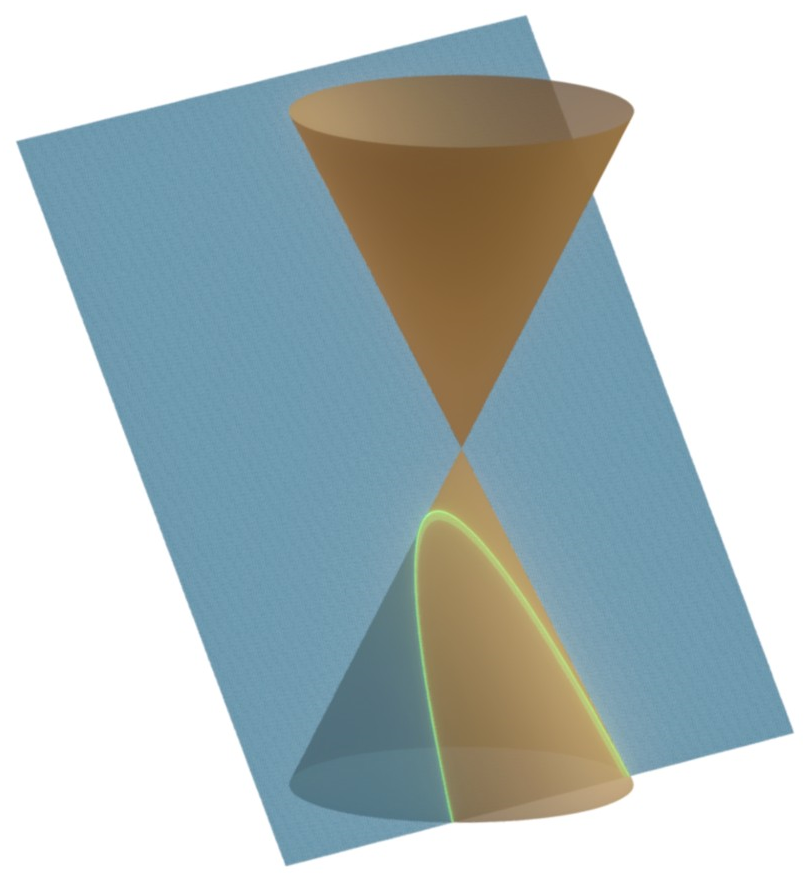
The last of our conic sections is the parabola, which is what we get when we slice through our double-cone parallel to the edge.

Wait, really? Parabolas?

Like, those parabolas, the kind that look like the graph of \(y=x^2\)?

Remember that conic sections were studied as far back as ancient Greece, long before anyone had thought of using algebraic equations to study them. So imagine René Descartes's surprise when he realized that the equation \(y=x^2\) produced this well-known shape! (You might take that for granted, but remember, somebody had to discover all these things!)

And knowing that a parabola is a kind of conic section means that there's a lot more we can learn about them than you might have originally thought. That's one of the great things about math — there are always new insights to be found.

The "anatomy" of a parabola is particularly interesting.

Drag the black point around.
Notice the two distances are always the same.

• Instead of having two foci, a parabola is generated by a single focus and a line called the directrix. The key feature is that for any point on the parabola, its distance to the focus is always the same as its distance from the directrix.
• The "turning point" of the parabola, which is closest to the focus, is called the vertex. The distance from the vertex to the focus is called the focal length.
• There's also an important chord that goes through the focus and is parallel to the directrix, called the latus rectum. The latus rectum is particularly useful in drawing the parabola, as we'll see in class.

Just like with the other conic sections, the focus of a parabola has a useful property: If a ray is emitted from the focus and bounces off the parabola, it will be always be parallel to the axis of symmetry.

Drag the black point around to see the reflection.

Preview Activity 21

Answer these questions and submit your answers as a document on Moodle. (Please submit as .docx or .pdf if possible.)

1. Open the following link in Desmos:

   https://www.desmos.com/calculator/nqggv7ppu4

   Play with the graphs by turning the horizontal and vertical parabola folders on and off, and dragging the points around.

   What observations can you make about how the shape of the parabola changes as you do so?

2. In the same Desmos window, graph the parabola with the following equation: \[y-4=\dfrac14(x+2)^2\] Then find a way to move around one of the points to get it to match this parabola.
   1. What is the vertex?
   2. What is the focus?
   3. What is the equation of the directrix?
   4. What is the focal length, and what is the length of the latus rectum?
3. In the same Desmos window, graph the parabola with the following equation: \[x+2=-\dfrac18(y-3)^2\] Then find a way to move around one of the points to get it to match this parabola.
   (You might want to turn off the parabola from #2 so things don't get cluttered.)
   1. What is the vertex?
   2. What is the focus?
   3. What is the equation of the directrix?
   4. What is the focal length, and what is the length of the latus rectum?
4. Based on your answers to the previous two problems, how can you tell from the equation of a parabola whether it's going to be horizontal or vertical?
5. Answer AT LEAST one of the following questions:
   1. What was something you found interesting about this activity?
   2. What was an "a-ha" moment you had while doing this activity?
   3. What was the muddiest point of this activity for you?
   4. What question(s) do you have about anything you've done?

---

Return to MAT 130 main page

Posted by Bill Shillito